Poisson Distribution | Probability and Stochastic Process
Poisson distribution is applicable to discrete random variables. A discrete random variable X is said to follow Poisson distribution if it has:
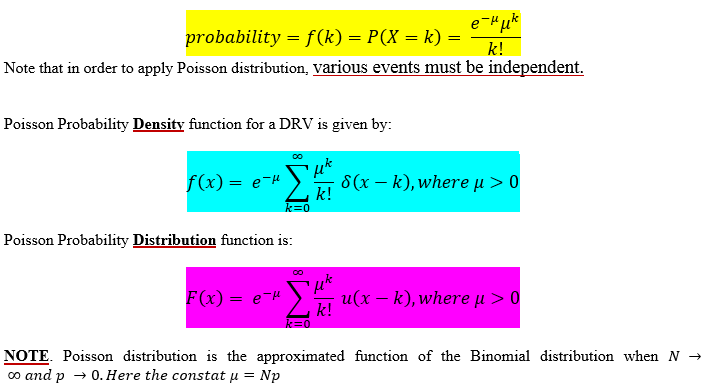
Note that in order to apply Poisson distribution, various events must be independent. Poisson RV is a count of number of events that occur in a certain time-interval. Examples are:
- Number of calls received during a given period of time
- Number of cars passing a fixed point in 5 minutes interval
- Number of customers visited during 1 PM – 2 PM
This Poisson random variable has wide spread applications such as:
- Telephone traffic
- Random failure of equipment
- Number of customers of a store/supermarket
- Queuing theory and queueing networks
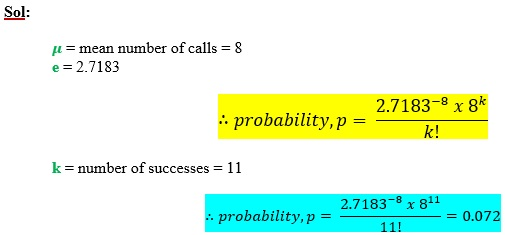
Q. Suppose the mean number of calls to a fire station on a week day is 8. What is the probability on a given
week day there would be 11 calls?
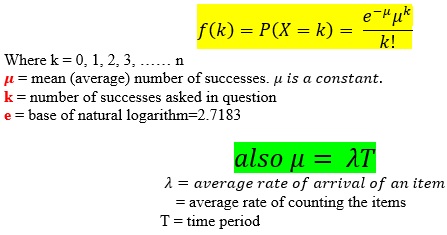
Poisson distribution is applicable to discrete random variables (DRVs). A discrete random variable X is said to follow Poisson distribution, if it has:
Applications:
- It is mostly applied to counting type of problems.
- Number of telephone calls made during a period of time
- Number of defective elements in a given sample
- Number of electrons emitted from a cathode in a given time interval
- Number of people waiting in a queue etc.