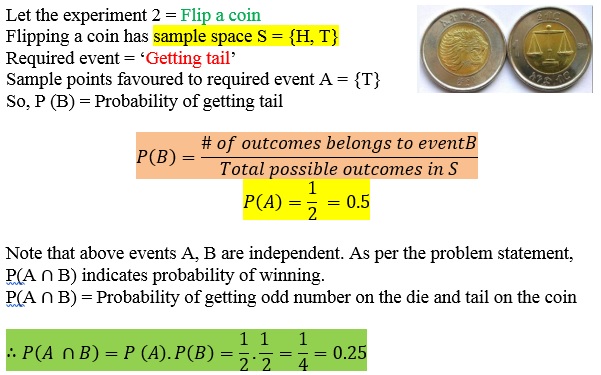
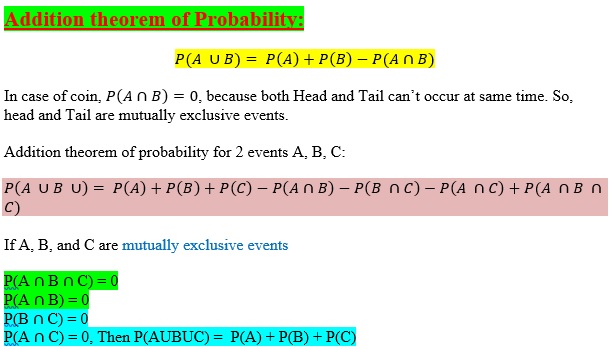
Types of Events | Probability Theory
Types of events
Event is a subset of the sample space. So an event may be a single element or set of elements of a sample space.
- Mutually Exclusive
- Equally Likely
- Independent
- Complementary Events
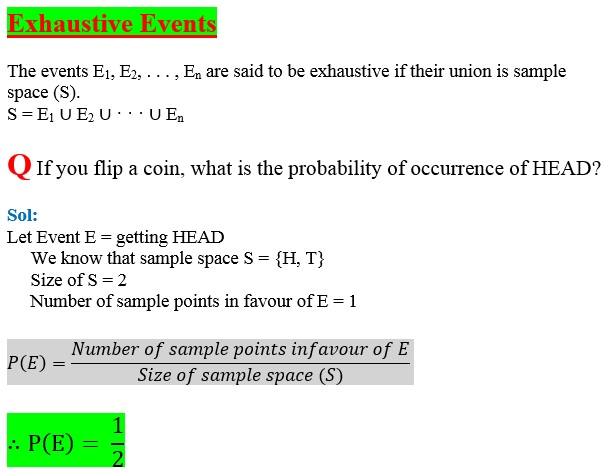
- Exhaustive
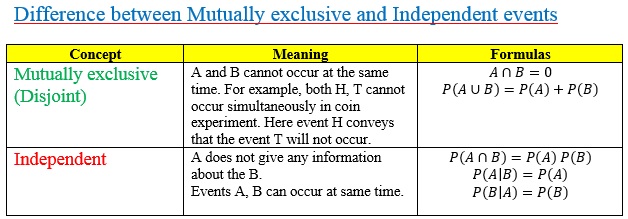
Mutually Exclusive Events
Two or more events are said to be mutually exclusive if occurrence of any one of them prevents the occurrence of all others.
Three mutually exclusive events: A, B, C are shown in below figure:
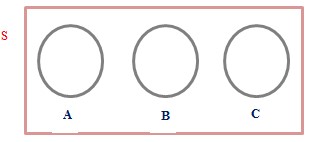
S = Sample Space
Note that the events are said to be mutually exclusive if they do not have any outcomes (elements) in common. Mutually exclusive events are also known as statistically independent events.
Example 1: Coin toss
When a coin is tossed, occurrence of Head prevents the occurrence of Tail. So, Head and tail mutually exclusive events.
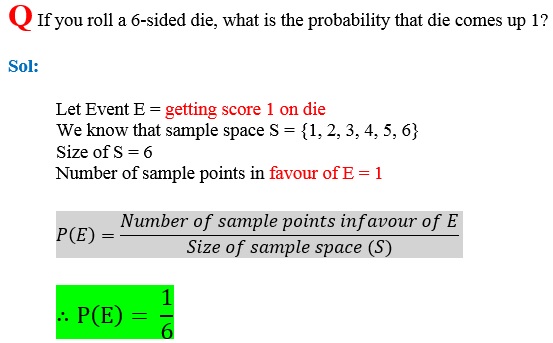
Example 2: Die throw
When a fair die is thrown, occurrence of #4 prevents the occurrence of other numbers 1, 2, 3, 5, 6. Note that ‘no two/more events can occur simultaneously’.

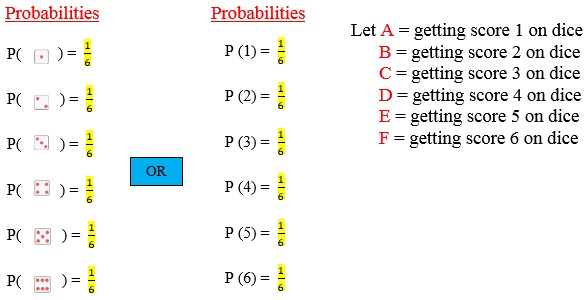
Observe that P(A) = P(B) = P(C) = P(D) = P(E) = P(F) =1/6. So, the events A, B, C, D, E, F events are said to be equally likely.
Example 2: Coin toss
Let A = {getting Head}
B = {getting Tail}
Size of sample space = 2
Observe that P(A) + P(B) = ½. Both the events have same probability.